Yamaha Montage 3 Synth Engines

Many people think of the Yamaha Montage as an FM synth and a sample based (AWM) synth.  But if you own an FM synth like the Yamaha Montage then you also own an synth with additive synthesis capabilities.

A typical waveform is the square wave.  A square wave is just all of the odd partials add 1/N amplitudes.   So, the fundamental has a relative amplitude of 1.0.  All even partials have a relative amplitude of 0 so we can skip those.  The 3^rd partial has a relative amplitude of 1/3 (0.33), the 5^th partial is 1/5 (0.20), the 7^th partial at (1/7) 0.14, the 9^th partial at (1/9) 0.11, the 11^th partial at 1/11 (0.09) , the 13^th at 1/13 (0.08), the 15^th at 1/15 (0.07) etc.

I used algorithm 1 which is all 8 operators as carriers.

Then set the relative frequency and levels as follows:

Operator	Frequency	Level
1	1.00	99
2	3.00	33
3	5.00	20
4	7.00	14
5	9.00	11
6	11.00	9
7	13.00	8
8	15.00	7

But the result sounded nothing like a square wave!  In fact, when I looked at the output, it looked like this:

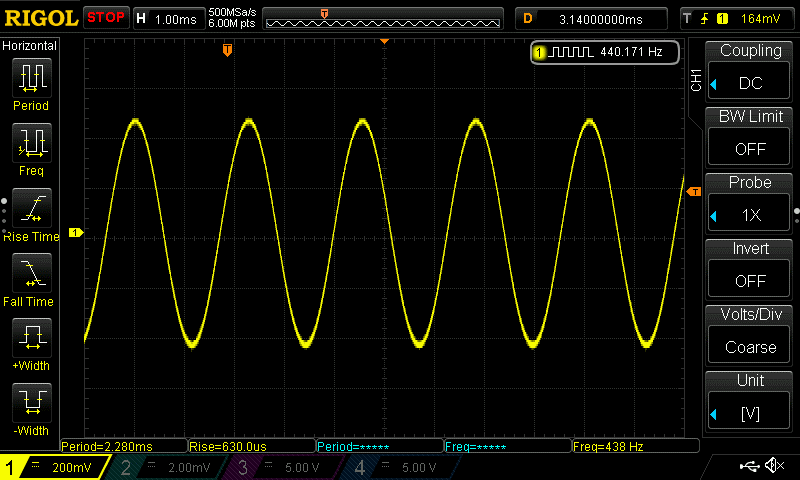
Which you can see is just a simple sine wave!

It turns out that the operator levels are not linear but exponential.  Here are the relative measurements I measured the AC voltage of the single operator output at 440Hz and normalized the results.

Op Lvl	Normalized Magnitude
99	100.000
98	92.000
97	84.400
96	77.300
95	70.900
94	65.290
93	59.880
92	54.920
91	50.360
90	46.190
89	42.360
88	38.840
87	35.610
86	32.650
85	29.940
84	27.460
83	25.180
82	23.080
81	21.150
80	19.400
79	17.790
78	16.310
77	14.950
76	13.710
75	12.560
74	11.520
73	10.560
72	9.670
71	8.860
70	8.130
69	7.450
68	6.820
67	6.250
66	5.730
65	5.250
64	4.810
63	4.400
62	4.040
61	3.700
60	3.390
59	3.100
58	2.840
57	2.600
56	2.380
55	2.180
54	2.000
53	1.830
52	1.670
51	1.530
50	1.400
49	1.280
48	1.180
47	1.070
46	0.980
45	0.900
44	0.820
43	0.750
42	0.690
41	0.630
40	0.570
39	0.520
38	0.480
37	0.430
36	0.390
35	0.360
34	0.330
33	0.300
32	0.270
31	0.250
30	0.220
29	0.200
28	0.180
27	0.170
26	0.150
25	0.140
24	0.120
23	0.110
22	0.100
21	0.090
20	0.080
19	0.060
18	0.050
17	0.040
16	0.030
15	0.030
14	0.030
13	0.020
12	0.020
11	0.020
10	0.020
9	0.010
8	0.010
7	0.010
6	0.010
5	0.010
4	0.010
3	0.010
2	0.010
1	0.010
0	0.000

At operator level 9 and lower, my DMM could not read any voltage so I just assumed it was a normalized level of about 0.01.  Now resetting the operator levels to the normalized values as follows:

Operator	Frequency	Level
1	1.00	99
2	3.00	86
3	5.00	80
4	7.00	76
5	9.00	74
6	11.00	71
7	13.00	69
8	15.00	68

Then I got something that looked like

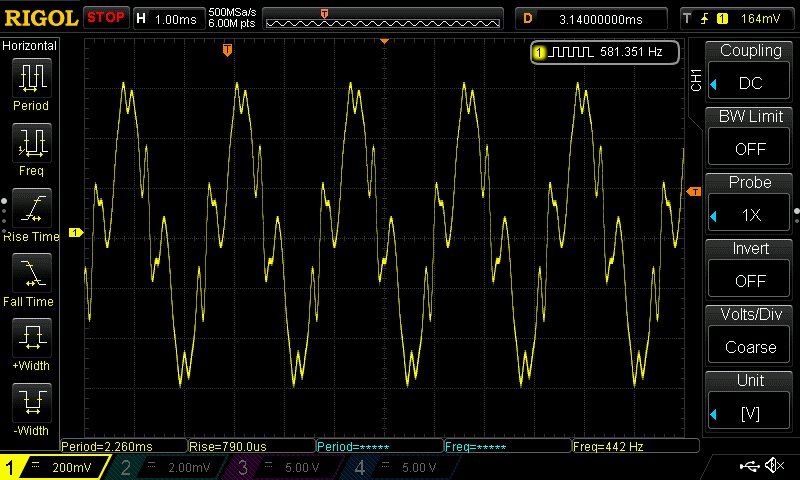
But that’s not a square wave – but it sounded like a square wave.  The scope output looked like the operators were not in phase.  After some investigation, I found that the operator frequencies drift.  I was playing A440 on the keyboard and the Montage is tuned to 440.0Hz.  I measured the frequency of a single sine wave on a DMM and found that the frequency would drift between 438Hz and 442Hz.  I also confirmed there is no pitch modulation on the operator.  I don’t know if this is intentional or an idiosyncrasy of the Montage OS version I am using which is version 3.50.0.  When I reset the oscilloscope to trigger at the beginning of the sound, it looked like

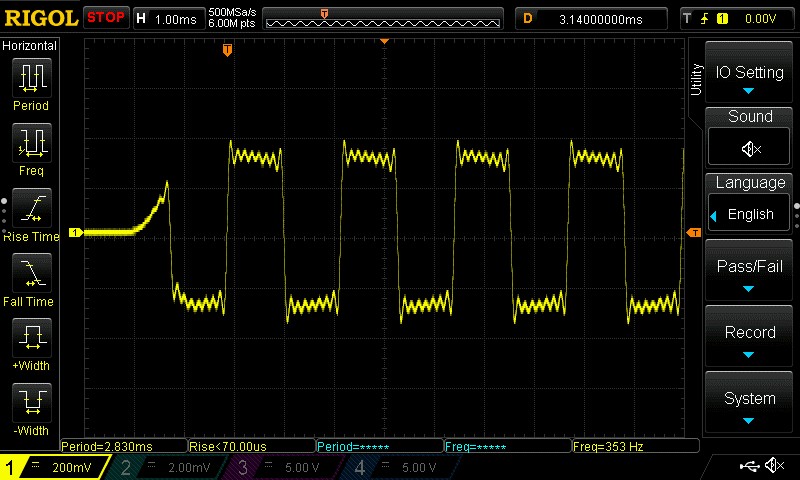
This looks very much like a square wave comprised of the first 8 partials.  So we can safely assume that the operators are in sync in the beginning but drifts over time.

The Montage can generate up to 31 partials, so let’s try that.   This can be accomplished with a performance with 2 FMX parts.

FMX Part 1

Operator	Frequency	Level
1	1.00	99
2	3.00	86
3	5.00	80
4	7.00	76
5	9.00	74
6	11.00	71
7	13.00	69
8	15.00	68

FMX Part 2

Operator	Frequency	Level
1	17.00	66
2	19.00	65
3	21.00	64
4	23.00	63
5	25.00	62
6	27.00	61
7	29.00	60
8	31.00	59

Here is what it looks like

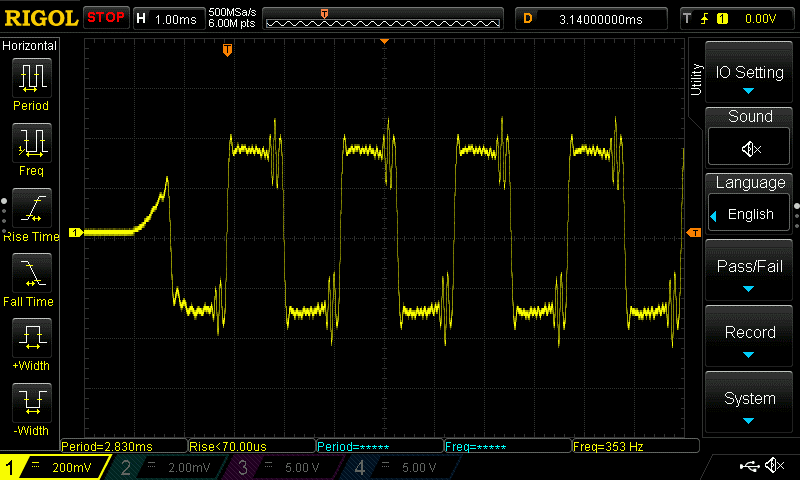
Is it a true square wave? No.  It has some very some “ringing” in wave which I cannot explain.  But when I compare it to the sampled square wave form (see below), it sounded the same to my ears.  I can’t hear much over 12kHz so it might sound different to you.

We also know that FM synthesis can generate a “square” wave with 2 operators stacked with a 2:1 Carrier/Modulator frequency ratio. 

Using any algorithm that has a 2 operator stack we can get square waves with varying degrees of “squareness”.  It’s not really a true square wave but get’s pretty close.  Here is the output of the waveform with various modulator operator output levels.

Modulator Output level is 67

Modulator Output Level 72

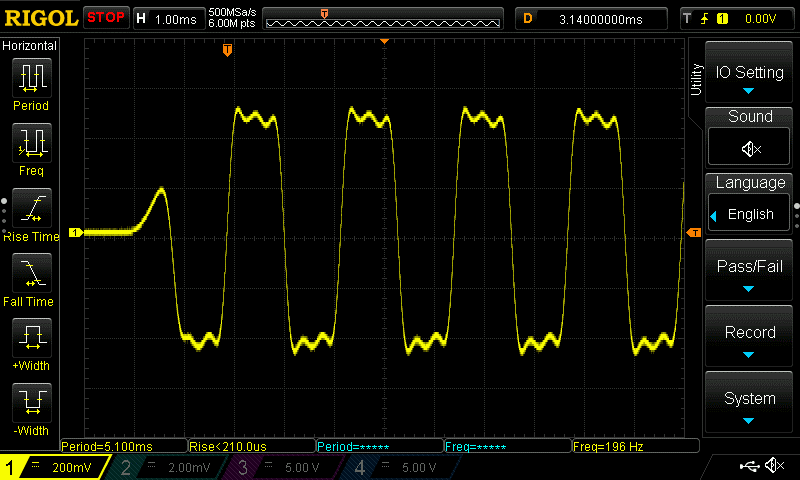
Modulator Output Level 75

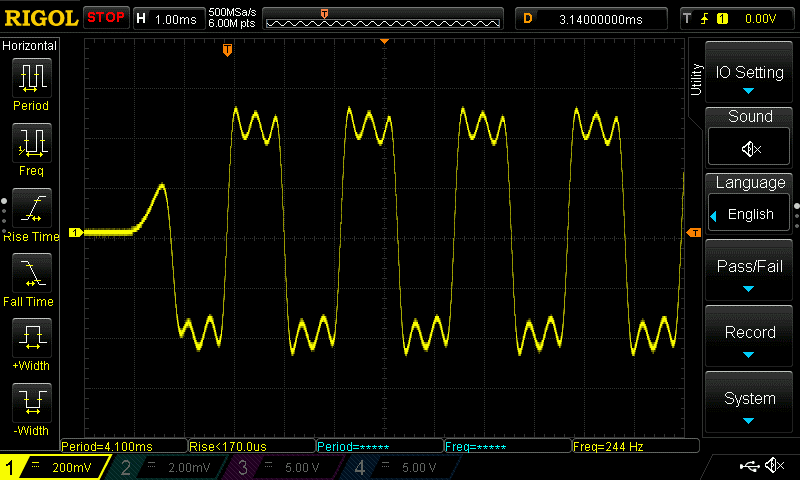
They look like square waves and sound like square waves but is not as bright as the squarewave generated with the additive synthesis.

The Montage has an AWM (sample based) synth engine also. If we look at the Synth waveforms, there is a square wave sample (#2136) called P50-1 which is a square wave with a 50% duty cycle and looks like.

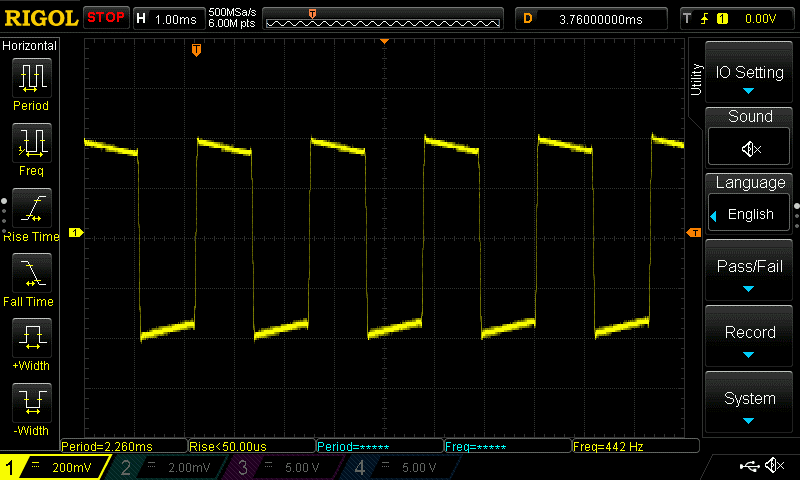
You can see it has the sharp pointy edges as you’d expect in a square wave but it had a bit of slope on the tops and bottoms which frankly surprised me.  Regardless, it sounded the same as the square wave created with additive synthesis.

So, the Montage has 3 unique ways to generate waveforms like a square wave.  Of course, the Montage has filters, effects, and a myriad of modulation sources which can be used to modulate these waveforms.